
By Dr. Pushpa Veerappan, Professor
Acoustics becomes much more complicated in indoor situations because sound energy has less volume in which to dissipate. Sound paths become more complicated as well. Any sound energy introduced into an enclosed space is subject to an almost infinite combination of modifiers, such as absorption, reflection, and summation. The relative proportions of each modifier can vary widely according to the listener location, with a corresponding effect on acoustical measurements.
Sound in a room is basically affected by two types of physical structures. The overall acoustics are influenced by room boundaries such as ceilings, floors and walls. Inconsistencies in the sound field can be created by in-room barriers,
Examples of room barriers would include large HVAC ducts, dividers, curtains, and balcony fronts.
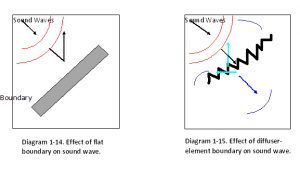
As shown in the diagrams, three things happen simultaneously and in varying proportions when sound strikes a boundary; transmission, absorption, and reflection. The proportion in which each happens is a function of the boundary material, size, mounting methodology, and the angle at which the sound strikes the boundary. Since we know that wavelength is a function of frequency, we might guess that the size of the boundary will have some effect on the frequencies reflected. All energy that is not reflected is either absorbed by the boundary and converted to heat, or passes through the boundary and is transmitted out the other side. Transmission and Absorption are frequency-selective. The mass, size, and density of the panel all affect the reflected, absorbed and transmitted spectrums.
Diagram 1-15 shows a side view of a specialized diffuser panel. The size of the indentations on the panel and the frequency response of the reflected energy is a result of a fairly complex correlation between the two. Acousticians use variations of this panel’s response-shaping reflective and diffusive capabilities to deliberately scatter certain frequencies in rooms such as recording studios and auditoriums.
The Reverberation Time Equation.
One popular acoustical treatment is absorption. Wallace Sabine, derived the relationship between boundary surfaces, room volume, mean free path (the average distance of sound before it strikes a boundary) and reverberation time.
The unit of absorption is named the “sabin” in honor of Dr. Sabine. His work is the foundation for modern acoustics. His equations have been modified since 1898 to allow for the effects of parallel surfaces and uneven absorption, but the original expression of the equation remains the standard for reverberation calculations.
Focused Sound.
A special set of problems arises when the room has unusual boundaries, such as a curved ceiling or a curved wall. This is particularly true in domed rooms. Domes may be thought of as boundaries curved in two dimensions.
Diffraction and Dispersion.
In addition to having reflective boundaries, a room may also have barriers that reflect, transmit, diffract, or disperse sound.
The determining factor is the frequency of the sound (which means its wavelength) in comparison to the size of the barrier. If the frequency wavelength is large in relation to the barrier, the waves will go around the barrier. If the barrier has a dimension facing the sound source that is equal to the wavelength, then the sound diffracts around the edges of the object. The exact behavior of the sound is dependent upon the angle of incidence and the shape of the barrier. When the barrier is larger than the wavelength, an acoustic shadow may result.
When sound strikes a large barrier that has an opening smaller than the wavelength of the sound the opening acts like a re-radiator of sound to the space on the other side of the barrier. The dispersion of the sound is inversely proportional to the size of the opening. This is one reason why the smallest opening between adjacent performance spaces can transmit and disperse much more sound than seems possible.
Standing Waves
Under certain conditions, the reflected sound of a solid boundary can combine with the original sound to form a standing wave. In order for this to happen, the wave’s peak amplitudes (positive and negative values) must synchronize in physical space to create a stationary pattern of peak pressures and rarefaction. The reflected wave’s superimposition on the original signal is similar to a situation where two of signals are exactly in phase. In the case of standing waves, the reflective boundary is the second “source.”
As we might suspect, standing waves in rooms are a product of frequency wavelengths that bear some exact relationship to the major room dimensions. A 125 Hz tone would have a wavelength of over 9 feet, and would create a standing wave between any two major parallel walls that were multiples of 9 feet apart. A room with dimensions of 27 feet by 45 feet by 18 feet high would create standing waves of 125 Hz in many directions if the walls were reflective at that frequency.
This 125 Hz wave would manifest itself in pressure zones and null points. A listener standing in a maximum pressure area would hear exaggerated bass at 125 Hz, while a listener at the null point would hear very little sound at the same frequency. Exact multiples of 125 Hz, such as 250 Hz, 375 Hz, 500 Hz, etc, will also generate standing waves. Many performance spaces and control rooms are non-rectangular in order to reduce standing waves.
Axial modes tend to be the strongest because the reflective path is the shortest in relation to room size, and because two opposing surfaces are firing reflections at each other. Common axial modes would be between end walls, side walls, and the pair of floor-ceiling. This assumes that each set is composed of parallel pairs. Room modes are functions of the relationships between room dimensions and frequency wavelengths
The Haas or precedence effect
It is used by sound system designers when a supplemental time-delay loudspeaker must be used to overcome adverse acoustics or blockage of the main speaker. This effect considers the ears inability to determine the location of a sound from two sources if it arrives at the ear close to the same time. For example, listeners under a balcony are sometimes not in a direct line-of-sight path of the overhead stage speakers, with the result that the high frequencies and intelligibility are both reduced for these listeners. The solution to this problem is to put speakers under the balcony, with the same signal as the main speakers, but with a time delay slightly longer than the time it takes for the main speaker energy to arrive under the balcony. By increasing the delay time of the remote speaker signal up to 20 milliseconds over the acoustic signal from the main cluster, the level of the remote speaker may be raised up to 10 dB above the main speaker level without the listeners being aware of the signal as coming from the remote speakers. High frequencies, and intelligibility, are restored with the use of these speakers.
Intelligibility
The ultimate design objective for most sound systems is the transmission of spoken information in such a way that the listeners understand every word. The degree to which this is accomplished is called the intelligibility percentage of the system. It is equal to the percentage of words a large group of randomly seated listeners would get correct on a standardized word-list test broadcast over the sound system. Sound system power, noise, distortion, frequency response and loudspeaker radiation characteristics can affect the intelligibility scores as much or more than the acoustical environment. Acoustical factors affecting intelligibility include ambient and crowd noise, early decay rate, reverberation, reflections, adjacent speaker interference, and intrusive sounds from outside the immediate area.
Perhaps the simplest and most widely used prediction method for intelligibility, was developed by a Dutch acoustician, V.M.A. Peutz, as a result of extensive word tests involving listeners at varying distances in a series of rooms with different reverberation times. Peutz found that intelligibility decreases with increasing source-to-listener distance up to the critical distance, after which it remains constant. The %ALCONS method (Articulation Loss of Consonants) calculates the number of consonants that will be misunderstood under specified conditions. Two predictive equations were developed, for distances less than or more than critical distance. The simplest form of the first is given below. In the real world, there are several more terms in the equation that deal with the amount of total loudspeaker array power reaching the listener and any special architectural (acoustic or electroacoustic) modifiers.
Intelligibility and Critical Distance.
The critical distance is the point at which the direct field from the loudspeaker equals the reverberant field produced by the room. Moving farther away from the loudspeaker the reverberant field stays constant, but intelligibility does not. Depending on the room, intelligibility may drop to unacceptable levels at between two and three times the critical distance from the loudspeakers. Rooms with long reverberation times (above 1.6 seconds in the bass and midbands) exhibit a more rapid falloff of intelligibility beyond the critical distance.
As a practical matter, the experienced sound system designer recognizes two realities: Critical distance is affected by two factors, one being the directivity of the loudspeaker and the other being the absorption of the room. It is much less expensive in most cases to increase the directivity of the loudspeakers and keep the sound away from reflective sources than it is to remodel the room. Critical distance changes with frequency, like reverberation time and the coverage angles of loudspeakers. A successful sound system design takes into account all three variables.
Masking
Any sort of sustained auditory interference from something not the original signal is called masking. Sources of masking may be air conditioners, air ducts, machinery noise, traffic, and so on. Since the most important intelligibility information is contained in the 2 kHz octave band, masking noise containing a significant amount of energy around 2 kHz will cause worsening of intelligibility as the noise level approaches the output level of the sound system.
As seen, enclosed spaces may create complex acoustic environments with equally complex sound fields. The idea is to control the above phenomena by considering the shape of the rooms and their finishing materials, in order to produce an acceptable acoustical environment in that given space.



